Physics 722

ASTROPHYSICS
Susan Lea
Spring 2006

Class schedule  
Date Assignment due
 Topic Book reference



R&L Shu Shore
Tu Jan 31
 none Radiation basics  1.1-5  Ch 1,2,3 Ch 3 , 6.2.4,5
Th Feb 2
 #1 Radiation basics
1.1-5
Tu Feb 7 #2  Einstein coefficients
Scattering
1.1-5  Ch 1,2,3 Ch 3 , 6.2.4,5
Th Feb 9
 Problem set 1 Atmospheres 1-6-8 Ch 1,2,3 Ch 3 , 6.2.4,5
Tu Feb 14
 #3 Larmor formula for radiation by accelerating charges Ch 2 Ch 11-14 Ch 3 , 6.2.4,5
Th Feb 16
Bremsstrahlung, Thomson scattering  3.1-4 Ch 11-14 Ch 3 , 6.2.4,5  §3.5.6
Tu Feb 21
Line emission
Line broadening; Curve of growth
relevant link 		  3.5-3.6 Ch2 §1-30 Ch 3 §3.3, 3.5.2-3.5.4
***** Paper topic statement due 2/21 *****
Th Feb 23 Problem set 2 Masers
Fluorescence		 Ch 7 Ch 14 Ch3 §3.5.6
Tu Feb 28
Compton scattering
Kompaneets equation 		 Ch 7  Ch 14
Th Mar  2
Kompaneets equation


Tu Mar 7
Synchrotron radiation Ch 6
Ch 6 § 6.5
Ch 7 § 7.7.2
Th Mar 9
 Problem set 3 Fluids
Vol  II
Ch 1-4		 Ch 1 § 1.4
Ch 1 § 1.5
Ch 4 § 4.2
Tu Mar 14
Virial theorem

VII Ch 8 Ch 1 § 1.4.4
Ch 3 § 3.5.1
Th Mar 16
 Problem set 4 Instabilities
V II Ch 15 Ch 6 § 6.8, 9
Tu Mar 21
Instabilities


Th Mar 23
Shock waves
V II Ch 15 Ch 6 § 6.7.1
Ch 6 § 6.7.5-6
Tu Mar 28
Shock waves


***** Abstract and outline due 3/28 *****
Th Mar 30

Ionization fronts
VII Ch 20
 Ch 6 § 6.7.2-4
Tu Apr 4-7

Spring Break


Tu Apr 11
 Problem Set 5 Ionization fronts



Th Apr 13
The two-body problem and binaries
V II Ch 6 Ch 1 § 1.2.1
 Tu Apr 18
The two-body problem and binaries


Th Apr  20
 Problem set 6 The three-body problem
V II Ch 6 Ch 5 § 5.5-6
Tu Apr 25
Accretion and winds

Ch 3 § 3.7
Th Apr 27
Accretion and winds


****** First draft of paper due 4/27 *****
Tu May 2
Accretion and winds


Th May 4
Advising Day


Tu May 9
Accretion disks
V II Ch 7 Ch 6 § 6.7.2-4
Th May 11
 A little cosmology

Ch 6 § 6.7.2-4
 ******* Second draft of paper due 5/11 **********
Tu May 16
 A little cosmology

Ch 8
Th May 18
Student presentations
Last day of classes


**** Final paper due Tuesday 5/23 *****
Th May 25
Presentations continue


Assignments

PROBLEM SET 1 Due Thursday Feb 9
Shu Vol I P set 1 Problem #2

PROBLEM SET 2 Due Thursday Feb 23
Rybicki and Lightman Problems 1.3, 1.5, 1.10
4. A spherical cloud has diameter 10 pc and density 100 cm-3 and the material in it is completely ionized at temperature 106 K.
a) Calculate the optical depth through the center of the cloud to free free absorption.
b) Calculate the optical depth due to Thomson scattering.
c) What is the effective optical depth due to scattering and absorption?
d) Make a plot of taueff versus frequency . (Use a log/log plot.)  Comment on your results. Plot tauabs on the same graph and compare the two plots.
These numbers are good estimates for a typical supernova remnant.
Repeat the calculations and graphs for a model of an x-ray source with T = 108 K, n = 1016 cm-3, and d = 1010 cm.
Numbers in cgs units

PROBLEM SET 3 Due Thursday March 9

R&L Problem 3.5

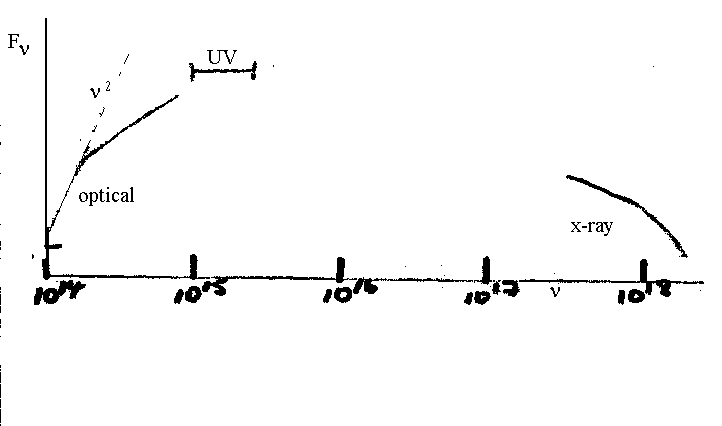
2. Scorpius X-1 is a very bright x-ray source which has a peculiar ("old-Nova-like") optical counterpart. A model has been developed for this source (eg Felton and Rees, 1972, A & A 17, 226) in which both the optical and x-ray emission emerge from the same cloud of ionized gas, which presumably surrounds and is heated by the central engine, whatever it might be. Use simple arguments based on the model described above, together with the data below,  to estimate the size and density of the emitting cloud.

Data for Sco X-1 (See diagram)
x-ray spectrum can be fit by a thermal bremsstrahlung spectrum with T = 7 x 107 K.
Fnu(1014 Hz) = 1.26 x 10-25 erg (cm2s.Hz)-1
Fnu(1015 Hz) = 1.5 x 10-24 erg (cm2s.Hz)-1
Fnu(3 keV) = 2 x 10-25 erg (cm2s.Hz)-1
scox-1


PROBLEM SET 4 Due Thursday March 16th
R&L Problem 6.4

PROBLEM SET 5 Due Thursday April 11th

1. The velocity dispersion (measured in radial velocity) for the Coma Cluster is about 1000 km/s.  (This is the rms velocity about the mean.)  To apply the virial theorem to the cluster, we convert this to a 3-D velocity dispersion by multiplying by the square root of 3.  (Explain why.)  The effective radius of the cluster is Reff = 220', and the redshift of the cluster is vr= 6888 km/s.  Assume that the gravitational energy of the cluster is just GM2/Reff .  Estimate the total mass of the cluster.  If the total luminosity is 1013 solar luminosities,  calculate M/L in solar units.  Comment on the results.  How do your answers depend on the Hubble constant?

2. Investigate the stability of a plane interface between two fluids of densities rho1  and rho2 .  Fluid 1 is moving with velocity v1 parallel to the boundary, and fluid 2 is moving with velocity v2 parallel to the boundary.  Use perturbation methods to analyse the evolution of a small displacement of the boundary under the following assumptions:

  1. incompressible flow
  2. zero gravity
  3. uniform equilibrium conditions
Here, as in the Rayleigh Taylor Instability, you'll find that you have to be very careful in finding the boundary conditions for the perturbed flow.
Give a physical explanation for your results.  This can be fairly "hand-waving".  Hint: Bernoulli's equation might be useful.
Discuss at least one astrophysical situation to which your result applies.

PROBLEM SET 6 Due Thursday April 20th
Shu Volume I Problem Set 3 #1.